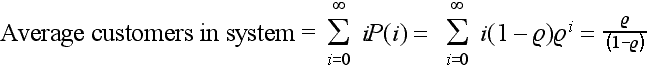M / M / 1 Model

With reference to the section on Kendall notation, the reader will realise that the M / M / 1 model is a queueing model where both the distribution of customer arrivals and the distribution of service times are assumed to be exponential, and there is a single server.

Definitions

• Let f(t) be the PDF of the inter-arrival times and let a be the average inter-arrival time.
• Let g(u) be the PDF of the service time and s be the average service time.
• Remember that both f(t) and g(u) are exponential distributions (see the section on statistics).
• Let  and  be as defined in the section on mathematics for all queueing models.

Probabilities

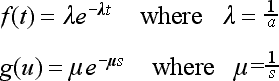
One can look at a small interval of time  and express P_t(i) after the small interval of time (the equations holds for an natural number i).

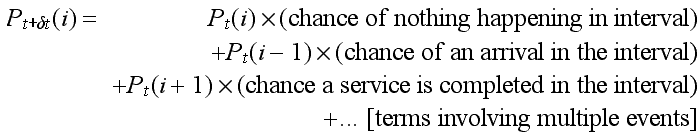
For small values of , the chance of a customer arrival to the queue is , and the chance of a service completion is . Thus the equation above can be solved as a differential equation as  tends to 0, with the correct values substituted in. The terms involving multiple events involve higher powers of , so they drop out during the analysis - demonstrating that multiple events cannot happen in a 'very short' period of time. The general solution to this equations is:

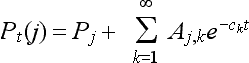
Also, it can shown from this that in a steady-state queueing system,

Server Utilisation

Customer Waiting Time

However, one can go one better than this and work out the PDF of the customer waiting time, so that more information can be found on how long a given customer may have to wait. It can be shown by consideration of the time the other customers will have to wait in the queue, and the most likely number of customers in the queue, that:

Average Number of Customers